Solution:
It is given that set of first ![]() natural numbers when
natural numbers when ![]() is an even number.
is an even number.
We now need to find the mean deviation about the mean
It is known that the first ![]() natural numbers are
natural numbers are ![]() . And given
. And given ![]() is even number.
is even number.
Therefore the mean is,
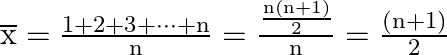
Deviations of numbers from the mean are as shown below,
![]()
![]()
Or, ![]()
Above eq. can be written as ![]()
Or,
![]()
Therefore, the absolute values of deviation from the mean is
![]()
Sum of absolute values of deviations from the mean, is ![]()
It can be written as
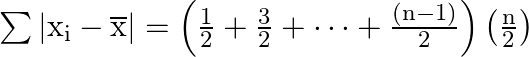
It is known that the sum of first ![]() natural numbers
natural numbers ![]()
Hence, the mean deviation about the mean is
M.D 
M. 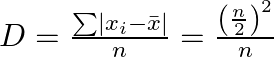
M. ![]()
As a result, ![]() is the mean deviation about the mean of the set of first
is the mean deviation about the mean of the set of first ![]() natural numbers when
natural numbers when ![]() is an even number.
is an even number.
