Login/Sign Up
Home » Consider a circular current-carrying loop of radius R in the x-y plane with centre at the origin. Consider the line integrala) show thatmonotonically increases with L b) use an appropriate Amperian loop to thatwhere I is the current in the wire c) verify directly the above result d) suppose we replace the circular coil by a square coil of sides R carrying the same current I. What can you say about
Consider a circular current-carrying loop of radius R in the x-y plane with centre at the origin. Consider the line integral\Im (L)=\left| \left. \int_{-L}^{L}{B.dl} \right| \right.a) show that\Im (L)monotonically increases with L b) use an appropriate Amperian loop to that\Im (\infty )={{\mu }_{0}}Iwhere I is the current in the wire c) verify directly the above result d) suppose we replace the circular coil by a square coil of sides R carrying the same current I. What can you say about\Im (\infty )\text{and }\Im \text{(L)}
CBSE | Class 12 | Moving Charges and Magnetism | ncert exemplar | Physics
Consider a circular current-carrying loop of radius R in the x-y plane with centre at the origin. Consider the line integral\Im (L)=\left| \left. \int_{-L}^{L}{B.dl} \right| \right.a) show that\Im (L)monotonically increases with L b) use an appropriate Amperian loop to that\Im (\infty )={{\mu }_{0}}Iwhere I is the current in the wire c) verify directly the above result d) suppose we replace the circular coil by a square coil of sides R carrying the same current I. What can you say about\Im (\infty )\text{and }\Im \text{(L)}

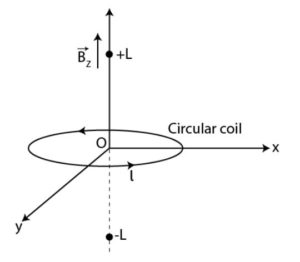
a) A circular current-carrying loop’s magnetic field is given as

\Im (L)=\int_{-L}^{+L}{Bdl}=2Bl

It is a L function that increases monotonically.

b) The Amperian loop is defined as follows:

\Im (\infty )=\int_{-\infty }^{+\infty }{\vec{B}dl}={{\mu }_{0}}I

c) The magnetic field at the circular coil’s axis is provided by 0I.

d) When a circular coil is swapped out for a square coil, the result is

\Im {{(\infty )}_{square}}=\Im {{(\infty )}_{\text{circular coil}}}

 

i

More Material