Login/Sign Up
Home » Determine the maximum value of     subject to the constraints:     .
Determine the maximum value of

    \[\mathbf{Z}\text{ }=\text{ }\mathbf{11}x~+\text{ }\mathbf{7}y~\]

subject to the constraints:

    \[\mathbf{2}x~+~y~\text{£}\text{ }\mathbf{6},~x~\text{£}\text{ }\mathbf{2},~x~{}^\text{3}\text{ }\mathbf{0},~y~{}^\text{3}\text{ }\mathbf{0}\]

.
CBSE | Class 12 | Exercise 1 | Linear Programming | Maths | NCERT Exemplar
Determine the maximum value of

    \[\mathbf{Z}\text{ }=\text{ }\mathbf{11}x~+\text{ }\mathbf{7}y~\]

subject to the constraints:

    \[\mathbf{2}x~+~y~\text{£}\text{ }\mathbf{6},~x~\text{£}\text{ }\mathbf{2},~x~{}^\text{3}\text{ }\mathbf{0},~y~{}^\text{3}\text{ }\mathbf{0}\]

.

According to the question:

    \[\mathbf{Z}\text{ }=\text{ }\mathbf{11}x~+\text{ }\mathbf{7}y~\]

and the constraints

    \[\mathbf{2}x~+~y~\text{£}\text{ }\mathbf{6},~x~\text{£}\text{ }\mathbf{2},~x~{}^\text{3}\text{ }\mathbf{0},~y~{}^\text{3}\text{ }\mathbf{0}\]

Let us consider

    \[2x\text{ }+\text{ }y\text{ }=\text{ }6\]

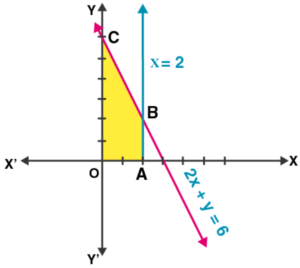
Now, plotting all the constrain equations we see that the shaded area OABC is the feasible region determined by the constraints.

The feasible region is bounded. So, the maximum value will occur at a corner point of the feasible region.

Corner points are

    \[\left( 0,\text{ }0 \right),\text{ }\left( 2,\text{ }0 \right),\text{ }\left( 2,\text{ }2 \right)\text{ }and\text{ }\left( 0,\text{ }6 \right)\]

On evaluating the value of Z, we get

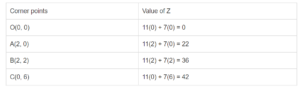
From the above table it’s seen that the maximum value of Z is

    \[42\]

.

Therefore, the maximum value of Z is

    \[42\]

at

    \[\left( 0,\text{ }6 \right)\]

.

i

More Material