Solution:
![]()
General solution:
For the differential equation in the form of ![]()
General solution is given by,
![]()
Where, integrating factor,
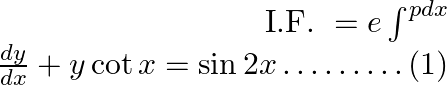
Equation (1) is of the form
![]()
Where, ![]() and
and ![]()
Therefore, integrating factor is
![]()
General solution is
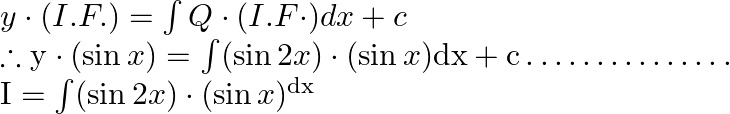
Let, ![]()
![]()
![]()
![]()
Again let, ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
Substituting I in (2),
![]()
Therefore, general solution is
![]()
