Solution:
It is known to us that the shortest distance between lines with vector equations ![]() and
and ![]() is given as
is given as
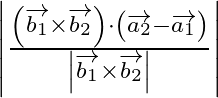
Given:
![]()
Let’s compare it with ![]() , we get
, we get ![]()
In the similar way,
![]()
Let’s compare it with ![]() , we obtain
, we obtain ![]()
Now,
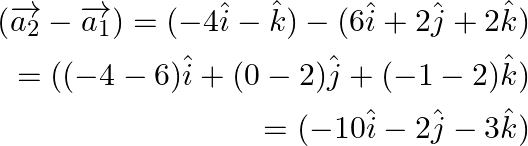
And,
![Rendered by QuickLaTeX.com \begin{array}{l} \left(\overrightarrow{b_{1}} \times \overrightarrow{b_{2}}\right)=\left|\begin{array}{ccc} \hat{i} & \hat{j} & \hat{k} \\ 1 & -2 & 2 \\ 3 & -2 & -2 \end{array}\right| \\ \left(\overrightarrow{b_{1}} \times \overrightarrow{b_{2}}\right)=\left|\begin{array}{ccc} \hat{i} & \hat{j} & \hat{k} \\ 1 & -2 & 2 \\ 3 & -2 & -2 \end{array}\right| \\ =\hat{i}[(-2 \times-2)-(-2 \times 2)]-\hat{j}[(1 \times-2)-(3 \times 2)]+\hat{k}[(1 \times-2)-(3 \times-2)] \\ =\hat{i}[4+4]-\hat{j}[-2-6]+\hat{k}[-2+6] \end{array}](https://www.learnatnoon.com/s/wp-content/ql-cache/quicklatex.com-b6d05a4d9dd18ecb2bff27afc0c7fd5c_l3.png)
![]() So, Magnitude of
So, Magnitude of 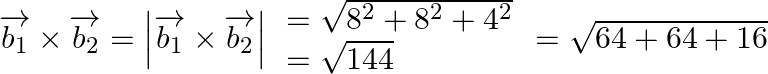
![]()
![]()
Also,
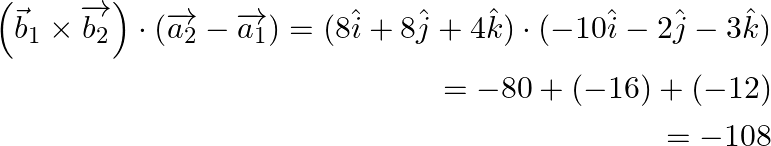
Therefore the shortest distance is given as
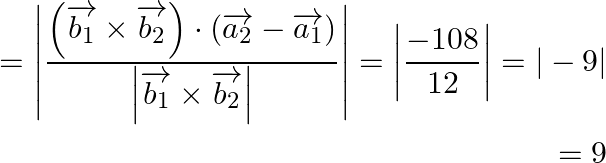
As a result, the shortest distance between the given two lines is 9 .
