Solution:
Given that ![]()
![]()
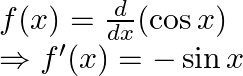
Taking different region from 0 to ![]()
Suppose ![]()
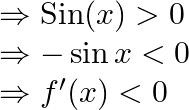
Therefore ![]() is decreasing in
is decreasing in ![]()
Suppose ![]()
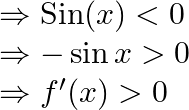
Therefore ![]() is increasing in
is increasing in ![]()
Thus, from above condition we find that
![]() is decreasing in
is decreasing in ![]() and increasing in
and increasing in ![]() .
.
As a result, condition for ![]() neither increasing nor decreasing in
neither increasing nor decreasing in ![]() ,
,
