A game consists o f tossing a 1 rupee coin three times, and noting Its outcomes each time. Find the probability o f getting (I) 3 heads, (II) at least 2 tails.
All kings, queens, and aces are removed from a pack o f 52 cards. The remaining cards are well-shuffled and then a card Is drawn from I t Find the probability that the drawn card Is
(I) a black face card,
(II)a red face card.
All red face cards are removed from a pack o f playing cards. The remaining cards are well-shuffled and then a card Is drawn at random from them. Find the probability that the drawn card Is
(I) a red card,
(II) a face card,
(III)a card of clubs.
What Is the probability that an ordinary year has 53 Mondays?
A card Is drawn at random from a well-shuffled pack o f 52 cards. Find the probability that the card was drawn Is neither a red card nor a queen.
5 cards the ten, Jack, queen, king and ace o f diamonds are well shuffled with their faces downward. One card Is then picked up at random. (a) What Is the probability that the drawn card Is the queen? (b) If the queen Is drawn and put aside and a second card Is drawn, find the probability that the second card Is (I) an ace, (II) a queen.
A letter Is chosen at random from the letter o f the word ‘ASSOCIATION’. Find the probability that the chosen letter Is a (I) vowel (II) consonant (III) S
Two dice are rolled once. Find the probability o f getting such numbers on 2 dice whose product Is a perfect square.
A die Is rolled twice. Find the probability that _9_ _ 3 12 4
(I) 5 w ill not come up either time,
(II) 5 w ill come up exactly one time,
(III) 5 w ill come up both the times.
A group consists o f 12 persons, o f which 3 are extremely patient, other 6 are extremely honest and rest are extremely kind. A person from the group Is selected at random. Assuming that each person Is equally likely to be selected, find the probability o f selecting a person who Is
(I) extremely patient,
(II) extremely kind o r honest. Which o f the above values did you prefer more?
A carton consists o f 100 shirts o f which 88 are good and 8 have minor defects. Rohlt, a trader, w ill only accept the shirts which are good. But, Kamal, and another trader w ill only reject the shirts which have major defects. 1 shirt Is drawn at random from the carton. What Is the probability that It Is acceptable to
(I)Rohlt,
(II) Kamal?
A Jar contains 54 marbles, each o f which some are blue, some are green and some are white. The probability o f selecting a blue marble at random Is and the probability o f selecting a green marble at random Is | . How many white marbles does the Jar contain?
A Jar contains 24 marbles. Some o f these are green others are blue. If a marble Is drawn at random from the Jar, the probability that It Is green Is | . Find the number o f blue marbles In the Jar.
A bag contains 18 balls out o f which x balls are red.
(I)If one ball Is drawn at random from the bag, what Is the probability that It Is not red?
(II) If two more red balls are put In the bag, the probability o f drawing a red ball w ill be | times the probability o f drawing a red ball In the firs t case. Find the value o f x.
The probability o f selecting a red ball at random from a Jar that contains only red, blue and orange balls Is j . The probability of selecting a blue ball at random from the same Jar Is j .If the Jar contains 10 orange balls, find the total number o f balls In the Jar.
A piggy bank contains hundred 50-p coins, seventy Rs. 1 coin, fifty Rs. 2 coins and th irty Rs. 5 coins. If It Is equally likely that one of the coins will fall out when the blank Is turned upside down, what Is the probability that the coin(I) will bea R s. 1 coin? (II) will not be a Rs. 5 coin (III) will be 50-p or a Rs. 2 coin?
A box contains 80 discs, which are numbered from 1 to 80. If one disc Is drawn at random from the box, find the probability that It bears a perfect square number.
Tickets numbered 2 ,3 ,4 , 5……………100,101 are placed In a box and mix thoroughly. One ticket Is drawn at random from the box. Find the probability that the number on the ticket Is(III) a number which Is a perfect square (Iv) a prime number less than 40.
Tickets numbered 2 ,3 ,4 , 5……………100,101 are placed In a box and mix thoroughly. One ticket Is drawn at random from the box. Find the probability that the number on the ticket Is
(I)an even number
(II)a number less than 16
Cards marked with numbers 1,3, 5……………..101 are placed In a bag and mixed thoroughly. A card Is drawn at random from the bag. Find the probability that the number on the drawn card Is
(I)less than 19,
(II) a prime number less than 20.
A box contains cards bearing numbers 6 to 70. If one card Is drawn at random from the box, find the probability that It bearsA box contains cards bearing numbers 6 to 70. If one card Is drawn at random from the box, find the probability that It bearsA box contains cards bearing numbers 6 to 70. If one card Is drawn at random from the box, find the probability that It bears
(III) an odd number less than 30,
(Iv) a composite number between 50 and 70.
A box contains cards bearing numbers 6 to 70. If one card Is drawn at random from the box, find the probability that It bears
(I) a 1 digit number,
(II)a number divisible by 5,
Cards bearing numbers 1,3, 5……………..35 are kept In a bag. A card Is drawn at random from the bag. Find the probability o f getting a card bearing
(I)a prime number less than 15,
(II) a number divisible by 3 and 5.
Card numbered 1 to 30 are put In a bag. A card Is drawn at random from the bag. Find the probability that the number on the drawn card Is
(I) not divisible by 3,
(II)a prime number greater than 7,
(III)not a perfect square number.
A box contains cards numbered 3, 5 , 7 , 9 ……..35,37. A card Is drawn at random from the box. Find the probability that the number on the card Is a prime number.
A box contains 25 cards numbers from 1 to 25. A card Is drawn at random from the bag. Find the probability that the number on the drawn card Is(I) divisible by 2 or 3, (II) a prime number.
A card Is drawn at random from a well-shuffled deck of playing cards. Find the probability that the card was drawn Is
(III)either a king or queen
(Iv) neither a king nor the queen.
A card Is drawn at random from a well-shuffled deck o f playing cards. Find the probability that the card was drawn Is
(I)a card of a spade or an Ace
(II)a red king
One card Is drawn from a well-shuffled deck of 52 cards. Find the probability o f getting(v) a Jack o f hearts (vl) a spade.
One card Is drawn from a well-shuffled deck of 52 cards. Find the probability o f getting
(III)a red face card
(Iv) a queen o f black suit
One card Is drawn from a well-shuffled deck of 52 cards. Find the probability o f getting
(I)a king o f red suit
(II) a face card
There are 40 students In a class o f whom 25 are girls and 15 are boys. The class teacher has to select one student as a class representative. He writes the name o f each student on a separate, the card being Identical. Then she puts cards In a bag and stirs them thoroughly. She then draws one card from the bag. What Is the probability that the name written on the card Is the name of
(I) A girl?
(II)A boy?
A bag contains lemon-flavored candles only. Hema takes out 1 candy without looking Into the bag. What Is the probability that she takes out
(I)An orange-flavored candy
(II)A lemon-flavored candy
(I) A lo t o f 20 bulbs contain 4 defective ones. 1 bulb Is drawn at random from the lot. What Is the probability that this bulb Is defective? (II) Suppose the ball drawn In
(I) Is not defective and not replaced. Now, ball Is drawn at random from the rest. What Is the probability that this bulb Is not defective?
A box contains 90 discs which are numbered from 1 to 90 If one disc Is drawn at random from the box, find the probability that It bears
(I) A two-digit number
(II) A perfect square number
(III) A number divisible by 5.
A lot consists o f 144 ballpoint pens o f which 20 are defective and others good. Tanvl will buy a pen If It Is a good but will not buy If It Is defective. The shopkeeper draws 1 pen at random and gives It to her. What Is the probability that
(I) She will buy It,
(II) She will not buy It?
12 defective pens are accidentally mixed with 132 good ones, It Is not possible to Just look at pen and tell whether or not It Is defective. 1 pen Is taken out at random from this lot. Find the probability that the pen taken out Is good one.
A game of chance consists of spinning and arrow which Is equally likely to come to the rest pointing to one of the numbers 1 , 2 ,3 ,4 12 as shown In the figure. What Is the probability that It will point to
(III)A prime number
(Iv) A number which Is a multiple o f 5
A game of chance consists of spinning and arrow which Is equally likely to come to the rest pointing to one of the numbers 1 , 2 ,3 ,4 12 as shown In the figure. What Is the probability that It will point to
(I)6
(II)An even number
Cards marked with numbers 5 to 50 are placed In a box and mixed thoroughly. A card Is drawn from the box at random. Find the probability that the number on the taken out card Is
Two dice are rolled together. Find the probability o f getting such numbers on the two dice whose product Is 12.
Two dice are rolled together. Find the probability o f getting such numbers on two dice whose product Is perfect square.
Solution: When two different dice are thrown, then total number of outcomes = 36. Let E be the event of getting the product of numbers, as a perfect square. These numbers are (1,1), (1,4), (2,2),...
When two dice are tossed together, find the probability that the sum o f the numbers on their tops Is less than 7.
Solution: When two different dice are thrown, the total number of outcomes = 36. Let E be the event of getting the sum of the numbers less than 7. These numbers are (1,1), (1,2), (1,3), (1,4),...
Two different dice are rolled simultaneously. Find the probability that the sum o f the numbers on the two dice Is 10.
Solution: When two different dice are thrown, the total number of outcomes = 36. Let E1 be the event of getting the sum of the numbers on the two dice is 10. These numbers are (4 ,6), (5,5)...
A coin is tossed. If it shows tail, we draw a ball from a box which contains 2 red 3 black balls; it shows head, we throw a die. Find the sample space of this experiment.
According to the question, A coin is tossed and there is box which contains 2 red and 3 black balls. When coin is tossed, the outcomes will be {H, T} According to question, if tail is turned up,...
An experiment consists of tossing a coin and then tossing it second time if head occurs. If a tail occurs on the first toss, then a die is tossed once. Find the sample space.
According to the question, a coin is tossed and if the outcome is tail then, a die will be rolled. The possible outcome for coin is 2 that is {H, T} And, the possible outcome for die is 6 that is...
A coin is tossed and then a die is rolled only in case a head is shown on the coin. Describe the sample space for this experiment.
According to the question, a coin is tossed and the die is rolled. So, when coin is tossed there will be 2 events that is either Head or tail, According to question, If Head occurs on coin then Die...
What is the total number of elementary events associated to the random experiment of throwing three dice together?
According to the question, three dice are thrown together. So there are 6 faces on die. As a result, the total numbers of elementary events on throwing three dice are $6^3=216$
Two dice are thrown. Describe the sample space of this experiment.
As we know, there are 6 faces on a dice containing (1, 2, 3, 4, 5, 6). According to the question, two dice are thrown, so we have two faces of dice (one of each). As a result, the total sample space...
Write the sample space for the experiment of tossing a coin four times.
According to the question, a coin is tossed four time, so the no. of samples will be, $2^4=16$ So, S = {HHHH, TTTT, HHHT, HHTH, HTHH, THHH, HHTT, HTTH, HTHT, THHT, THTH, TTHH, HTTT, THTT, TTHT,...
If a coin is tossed two times, describe the sample space associated to this experiment.
We know that if two coins are tossed, that means two probabilities will occur at same time. So, S = {HT, TH, HH, TT} ∴ Sample space is {HT, HH, TT, TH}
Choose the correct answer out of four given options in each of the exercise without repetition of the numbers, four digit numbers are formed with the numbers 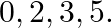 The probability of such a number divisible by 5 is
The probability of such a number divisible by 5 is
A. 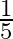
B. 
C. 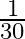
D. 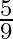
Solution: Option (D) $5 / 9$ Explanation: The digits $0,2,3,5 $ we have. It is known that, if unit place digit is ${ }^{\prime} 0^{\prime}$ or ${ }^{\prime} 5^{\prime}$ then the no. is divisible by...
Choose the correct answer out of four given options in each of the exercise seven persons are to be seated in a row. The probability that two particular persons sit next to each other is
A. 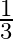
B. 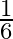
C. 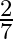
D. 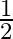
Solution: Option (C) $2 / 7$ is correct. Explanation: It is given that 7 persons are to be seated in a row. If two persons sit next to each other, then they'll be considered as 1 group. We now have...
Choose the correct answer out of four given options in each of the exercise while shuffling a pack of 52 playing cards, 2 are accidentally dropped. Find the probability that the missing cards to be of different colours
A. 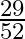
B. 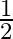
C. 
D. 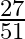
Solution: Option (C) $26 / 51$ is correct. Explanation: It is known that, in a deck of 52 cards 26 are red and 26 are of black. Given that 2 cards are accidentally dropped Therefore, Probability of...
Choose the correct answer out of four given options in each of the exercise three numbers are chosen from 1 to 20 . Find the probability that they are not consecutive
A. 
B. 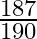
C. 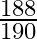
D. 
Solution: Option (B) $187 / 190$ is correct. Explanation: As, the set of 3 consecutive nos. from 1 to 20 are $(1,2,3),(2,3,4),(3,4,5), \ldots,(18,19,20)$ Consider the 3 numbers as a single digit...
Determine the probability 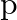 , for each of the fonowing,
, for each of the fonowing,
(a) The sum of 6 appears in a single toss of a pair of fair dice.
Solution: (a) When a pair of dice is rolled, total number of cases $\begin{array}{l} S=\{(1,1),(1,2),(1,3),(1,4),(1,5),(1,6) \\ (2,1),(2,2),(2,3),(2,4),(2,5),(2,6) \\...
Determine the probability 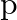 , for each of the fonowing,
, for each of the fonowing,
(a) An odd number appears in a single toss of a fair die.
(b) At least one head appears in two tosses of a fair coin.
Solution: (a) When a fair die is thrown, the possible outcomes are $S=\{1,2,3,4,5,6\}$ $\therefore$ Total no. of outcomes $=6$ and the odd numbers are $1,3,5$ $\therefore$ Favourable no. of outcomes...
A sample space consists of 9 elementary outcomes 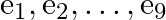 whose probabilities are
whose probabilities are 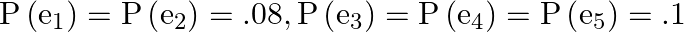
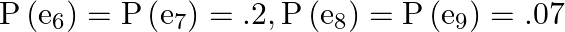 Suppose
Suppose 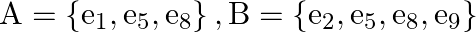
(a) List the composition of the event A U B, and calculate P (A U B) by adding the probabilities of the elementary outcomes.
(b) Calculate P (B) from P (B), also calculate P (B  directly from the elementary outcomes of
directly from the elementary outcomes of  .
.
Solution: It is given that $\begin{array}{l} S=\left\{e_{1}, e_{2}, e_{3}, e_{4}, e_{5}, e_{8}, e_{7}, e_{8}, e_{9}\right\} \\ A=\left\{e_{1}, e_{5}, e_{8}\right\} \text { and } B=\left\{e_{2},...
A sample space consists of 9 elementary outcomes 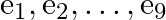 whose probabilities are
whose probabilities are 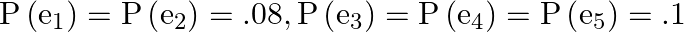
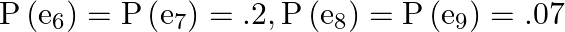 Suppose
Suppose 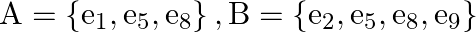
(a) Calculate  , and
, and 
(b) Using the addition law of probability, calculate P (A U B)
Solution: It is given that $\begin{array}{l} S=\left\{e_{1}, e_{2}, e_{3}, e_{4}, e_{5}, e_{8}, e_{7}, e_{8}, e_{9}\right\} \\ A=\left\{e_{1}, e_{5}, e_{8}\right\} \text { and } B=\left\{e_{2},...
A card is drawn from a deck of 52 cards. Find the probability of getting a king or a heart or a red card.
Solution: Total no. of playing cards $=52$ $\therefore n(S)=52$ Total king cards $=4$ Total heart cards $=13$ Total red cards $=13+13=26$ $\therefore$ No. of favourable outcomes $=4+13+26-13-2=28$...
If the letters of the word ASSASSINATION are arranged at random. Find the Probability that
(a) All A’s are not coming together
(b) No two A’s are coming together.
Solution: The given word is ASSASSINATION Total no. of letters in ASSASSINATION $=13$ In the given word ASSASSINATION, there are 3A’s, 4S’s, 2I’s, 2N’s, 1T’s and 1O’s $\therefore$ The total no. of...
If the letters of the word ASSASSINATION are arranged at random. Find the Probability that
(a) Four S’s come consecutively in the word
(b) Two I’s and two N’s come together
Solution: The given word is ASSASSINATION Total no. of letters in ASSASSINATION $=13$ In the given word ASSASSINATION, there are 3A’s, 4S’s, 2I’s, 2N’s, 1T’s and 1O’s $\therefore$ The total no. of...
One urn contains two black balls (labelled  and
and 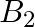 ) and one white ball. A second urn contains one black ball and two white balls (labelled
) and one white ball. A second urn contains one black ball and two white balls (labelled 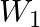 and
and 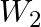 ). Suppose the following experiment is performed. One of the two urns is chosen at random. Next a ball is randomly chosen from the urn. Then a second ball is chosen at random from the same urn without replacing the first ball.
). Suppose the following experiment is performed. One of the two urns is chosen at random. Next a ball is randomly chosen from the urn. Then a second ball is chosen at random from the same urn without replacing the first ball.
(a) What is the probability that two balls of opposite colour are chosen?
Solution: (a) If two balls of opposite colours are chosen then The favourable outcomes are $\mathrm{B}_{1} \mathrm{~W}, \mathrm{~B}_{2} \mathrm{~W}, \mathrm{WB}_{1}, \mathrm{WB}_{2}, \mathrm{~W}_{1}...
One urn contains two black balls (labelled  and
and 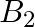 ) and one white ball. A second urn contains one black ball and two white balls (labelled
) and one white ball. A second urn contains one black ball and two white balls (labelled 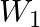 and
and 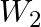 ). Suppose the following experiment is performed. One of the two urns is chosen at random. Next a ball is randomly chosen from the urn. Then a second ball is chosen at random from the same urn without replacing the first ball.
). Suppose the following experiment is performed. One of the two urns is chosen at random. Next a ball is randomly chosen from the urn. Then a second ball is chosen at random from the same urn without replacing the first ball.
(a) Write the sample space showing all possible outcomes
(b) What is the probability that two black balls are chosen?
Solution: It is given that one urn contains two black balls and one white ball and the second urn contains one black ball and two white balls. Also given that if one of the two urns is chosen, then...
The accompanying Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections (for instance, P (A ∩ B) = .07. Determine
(a) P (B ∩ C)
(b) Probability of exactly one of the three occurs.
Solution: It is given that $\mathrm{P}(\mathrm{A} \cap \mathrm{B})=0.07$ From the Venn Diagram given (a) $P(B \cap C)$ From the Venn diagram we have $P(B \cap C)=0.15$ (b) Probability of exactly one...
The accompanying Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections (for instance, P (A ∩ B) = .07. Determine
(a) P (A ∪ B)
(b) P (A ∩ B)
Solution: It is given that $\mathrm{P}(\mathrm{A} \cap \mathrm{B})=0.07$ From the Venn Diagram given (a) $P(A \cup B)$ Using the General Addition Rule, $P(A \cup B)=P(A)+P(B)-P(A \cap B)$ Substitute...
The accompanying Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections (for instance, P (A ∩ B) = .07. Determine
(a) P (A)
(b) 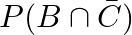
Solution: It is given that $\mathrm{P}(\mathrm{A} \cap \mathrm{B})=0.07$ From the Venn Diagram given (a) P(A) (b) $P(B \cap \bar{C})$ $P(B \cap \bar{C})=P(B)-P(B \cap C)$ Substitute the values,...
One of the four persons John, Rita, Aslam or Gurpreet will be promoted next month. Consequently, the sample space consists of four elementary outcomes S = {John promoted, Rita promoted, Aslam promoted, Gurpreet promoted} You are told that the chances of John’s promotion is same as that of Gurpreet, Rita’s chances of promotion are twice as likely as Johns. Aslam’s chances are four times that of John.
(a) Determine P (John promoted)
P (Rita promoted)
P (Aslam promoted)
P (Gurpreet promoted)
(b) If A = {John promoted or Gurpreet promoted}, find P (A).
Solution: Given that: Sample Space, S $=$ John promoted, Rita promoted, Aslam promoted, Gurpreet promoted Let's say Events that John promoted $E_{1}$ Events that Rita promoted $E_2$ Events that...
Four candidates A, B, C, D have applied for the assignment to coach a school cricket team. If A is nd C are given about the same chance of being selected, D, what are the probabilities that
(a) C will be selected?
(b) A will not be selected?
Solution: It is given that A is twice as likely to be selected as $B$ that is $P(A)=2 P(B) \ldots \ldots 1$ and $C$ is twice as likely to be selected as $D$. that is $P(C)=2 P(D) \ldots \ldots 2$ So...
A team of medical students doing their internship have to assist during surgeries at a city hospital. The probabilities of surgeries rated as very complex, complex, routine, simple or very simple are respectively, 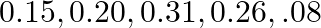 . Find the probabilities that a particular surgery will be rated.
. Find the probabilities that a particular surgery will be rated.
(a) routine or complex
(b) routine or simple
Solution: Let' say Event that surgeries are rated as very complex $=E_{1}$ Event that surgeries are rated as complex $=\mathrm{E}_{2}$ Event that surgeries are rated as routine $=\mathrm{E}_{3}$...
A team of medical students doing their internship have to assist during surgeries at a city hospital. The probabilities of surgeries rated as very complex, complex, routine, simple or very simple are respectively, 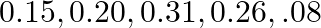 . Find the probabilities that a particular surgery will be rated.
. Find the probabilities that a particular surgery will be rated.
(a) complex or very complex;
(b) neither very complex nor very simple;
Solution: Let' say Event that surgeries are rated as very complex $=E_{1}$ Event that surgeries are rated as complex $=\mathrm{E}_{2}$ Event that surgeries are rated as routine $=\mathrm{E}_{3}$...
If A and B are mutually exclusive events, P (A) = 0.35 and P (B) = 0.45, find
(a) P (A ∩ B′)
(b) P (A′∩ B′)
Solution: Given: $P(A)=0.35$ and $P(B)=0.45$ $\because$ The events $A$ and $B$ are mutually exclusive then $P(A \cap B)=0$ (a) We need to find $P\left(A \cap B^{\prime}\right)$ $\begin{array}{l}...
If A and B are mutually exclusive events, P (A) = 0.35 and P (B) = 0.45, find
(a) P (A ∪ B)
(b) P (A ∩ B)
Solution: Given: $P(A)=0.35$ and $P(B)=0.45$ $\because$ The events $A$ and $B$ are mutually exclusive then $P(A \cap B)=0$ (a) We need to find $P(A \cup B)$ It is known that, $\begin{array}{l} P(A...
If A and B are mutually exclusive events, P (A) = 0.35 and P (B) = 0.45,
find (a) P (A′) (b) P (B′)
Solution: Given: $P(A)=0.35$ and $P(B)=0.45$ $\because$ The events $A$ and $B$ are mutually exclusive then $P(A \cap B)=0$ (a) We need to find $P\left(A^{\prime}\right)$ It is known that,...
In a large metropolitan area, the probabilities are .87, .36, .30 that a family (randomly chosen for a sample survey) owns a colour television set, a black and white television set, or both kinds of sets. What is the probability that a family owns either anyone or both kinds of sets?
Solution: Event that a family owns colour television $=E_{1}$ Event that the family owns black and white television $=E_{2}$ Provided that $P\left(E_{1}\right)=0.87$ $P\left(E_{2}\right)=0.36$ and...
A die is loaded in such a way that each odd number is twice as likely to occur as each even number. Find P(G), where G is the event that a number greater than 3 occurs on a single roll of the die.
Solution: The probability of odd numbers $\begin{array}{l} =2 \times \text { (Probability of even number) } \\ \Rightarrow P(\text { Odd })=2 \times P \text { (Even) } \\ \text { Now, } P(\text {...
An experiment consists of rolling a die until a 2 appears.
(i) How many elements of the sample space correspond to the event that the 2 appears on the 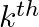 roll of the die?
roll of the die?
(ii) How many elements of the sample space correspond to the event that the 2 appears not later than the 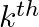 roll of the die?
roll of the die?
Solution: The given no. of outcomes when die is thrown $=6$ (i) If 2 appears on the $k^{th}$ roll of the die. Therefore, the first $(k-1)$ roll have 5 outcomes each and $K^{th}$ roll results 2 No....
Suppose an integer from 1 through 1000 is chosen at random, find the probability that the integer is a multiple of 2 or a multiple of 9.
Solution: We have integers $1,2,3, \ldots 1000$ We have integers $1,2,3, \ldots 1000$ $(S)=1000$ No. of integers that are multiple of $2=500$ Let's say the no. of integers that are multiple of 9 be...
Six new employees, two of whom are married to each other, are to be assigned six desks that are lined up in a row. If the assignment of employees to desks is made randomly, what is the probability that the married couple will have nonadjacent desks?
Solution: The total new employees $=6$ Therefore, they can be arranged in $6!$ Ways $\therefore \mathrm{n}(\mathrm{S})=6 !=6 \times 5 \times 4 \times 3 \times 2 \times 1=720$ In 5 ways two adjacent...
If the letters of the word ALGORITHM are arranged at random in a row what is the probability the letters GOR must remain together as a unit?
Solution: The given word is ALGORITHM $\Rightarrow$ The total no. of letters in algorithm $=9$ $\therefore$ The total no. of words $=9 !$ Therefore, $n(S)=9 !$ If 'GOR' remain together, then it will...
5. The percentage of marks obtained by a student in monthly unit tests are given below
Unit TestIIIIIIIVVVIPercentage of marks obtained by students$72$$67$$69$$74$$71$$76$ (iii) less than $65%$marks in a unit test. Answer- Probability – the chances of something happening. (iii) The...
The percentage of marks obtained by a student in monthly unit tests are given below
Unit Test$$IIIIII1VVVIPercentage of marks obtained$72$$67$$69$$74$$71$$76$ Based on this data find the probability that the student gets. (i) More than $70%$ marks in a unit test. (ii) less than...
A die is thrown 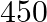 times and frequencies of the outcomes
times and frequencies of the outcomes 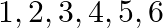 were noted as given in the following table
were noted as given in the following table
Outcomes$1$$2$$3$$4$$5$$6$Frequency$73$$70$$74$$75$$80$$78$ (iii) a number $>4$ SOLUTION- (iii) Number of times greater than 4 come on the die = $80+78=158$ $P(>4$will come up on die$)$...
A die is thrown 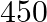 times and frequencies of the outcomes
times and frequencies of the outcomes 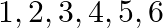 were noted as given in the following table
were noted as given in the following table
outcome$1$$2$$3$$4$$5$$6$Frequency$73$$70$$74$$75$$80$$78$ (i) $4$ (ii) a number $<4$ Solution:- In the question it is given that, a die is thrown $450$ times So, total number of times die thrown...
2. 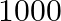 families with
families with 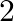 children were selected randomly, and the following data were recorded:
children were selected randomly, and the following data were recorded:
Number of girls in a family$0$$1$$2$Number of families$333$$392$$275$ Find the probability of a family, having (iii) no girl Solution:- The extent to which an event is likely to occur and measured...
2. 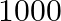 families with
families with 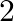 children were selected randomly, and the following data were recorded:
children were selected randomly, and the following data were recorded:
Number of girls in a family$0$$1$$2$Number of families$333$$392$$275$ Find the probability of a family, having (i) $1$ girl (ii) $2$ girls Solution:- Probability is the branch mathematics...
1. A coin is tossed 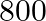 times and the outcomes were noted as: Head:
times and the outcomes were noted as: Head: 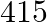 , Tail :
, Tail : 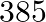 . Find the probability of the coin showing up (i) a head, (ii) a tail.
. Find the probability of the coin showing up (i) a head, (ii) a tail.
Probability means possibility. It is a branch of mathematics that deals with the occurrence of a random event. The value is expressed from zero to one. From the question it is given that, A...
Two dice are thrown simultaneously. If 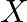 denotes the number of sixes, find the expectation of
denotes the number of sixes, find the expectation of 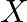 .
.
Solution: A die is tossed twice in this game. When a dice is thrown twice, the total number of observations is $(6 \times 6)=36$. Let $\mathrm{X}$ be a random variable that symbolises success and is...
Find the mean number of heads in three tosses of a fair coin.
Solution: Three times, a coin is tossed. Three coins are flung at the same time. As a result, the experiment's sample space is $\mathrm{S}=$ \{HHH, HHT, HTH, THH, TTH, THT, HTT, TTT\}. The number of...
In a meeting,  of the members favour and
of the members favour and 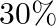 oppose a certain proposal. A member is selected at random and we take
oppose a certain proposal. A member is selected at random and we take 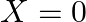 if he opposed, and
if he opposed, and 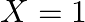 if he is in favour. Find
if he is in favour. Find 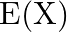 and
and 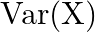 .
.
Solution: If members are opposed, $X=0$, and if members are in favour, $X=1$. $\mathrm{P}(\mathrm{X}=0)=30 \%=30 / 100=0.3$ $P(X=1)=70 \%=70 / 100=0.7$ As a result, the necessary probability...
The mean of the numbers obtained on throwing a die having written 1 on three faces, 2 on two faces and 5 on one face is
A. 1 B. 2 C. 5 D. $8 / 3$ Solution: B. 2 Explanation: A dice with the numbers 1 on three faces, 2 on two faces, and 5 on one face is given. Let $X$ represent a random variable that represents a...
Suppose that two cards are drawn at random from a deck of cards. Let 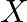 be the number of aces obtained. Then the value of
be the number of aces obtained. Then the value of 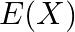 is A.
is A.  B.
B.  C.
C.  D.
D. 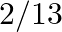
Solution: D. $2 / 13$ REASON: A deck of cards is given. Let X be the total number of aces you've gotten. Therefore Expectation of $\mathrm{X} \mathrm{E}(\mathrm{X})$...
A coin is biased so that the head is 3 times as likely to occur as tail. If the coin is tossed twice, find the probability distribution of number of tails.
head is 3 times as likely to occur as tail. Now, let the probability of getting a tail in the biased coin be x. ⇒ P(T) = x And P(H) = 3x For a biased coin, P(T) + P(H) = 1...
Let 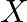 represent the difference between the number of heads and the number of tails obtained when a coin is tossed 6 times. What are possible values of
represent the difference between the number of heads and the number of tails obtained when a coin is tossed 6 times. What are possible values of 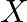 ?
?
Solution: Six times a coin is tossed. The difference between the number of heads and the number of tails is represented by X. $\Rightarrow \mathrm{X}(6 \mathrm{H}, \mathrm{OT})=|6-0|=6$ $X(5 H, 1...
If  and
and  are two events such that
are two events such that 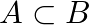 and
and 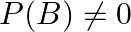 , then which of the following is correct?
, then which of the following is correct?
A. $P(A \mid B)=P(B) / P(A)$ B. $P(A \mid B)<P(A)$ C. $P(A \mid B) \geq P(A)$ D. None of these Solution: C. $P(A \mid B) \geq P(A)$ $A$ and $B$ are two events in which $A \subset B$ and...
Probability that 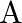 speaks truth is
speaks truth is 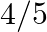 . A coin is tossed. A reports that a head appears. The probability that actually there was head is
. A coin is tossed. A reports that a head appears. The probability that actually there was head is
A. $4 / 5$ B. $1 / 2$ C. $1 / 5$ D. $2 / 5$ Solution: A. $4 / 5$ Explanation: Let $E_{1}$ represent the event in which A speaks the truth, $E_{2}$ represent the event in which A lies, and $X$...
A card from a pack of 52 cards is lost. From the remaining cards of the pack, two cards are drawn and are found to be both diamonds. Find the probability of the lost card being a diamond.
Solution: Let E1 represent the probability that the drawn card is a diamond, E2 represent the probability that the drawn card is not a diamond, and $\mathrm{A}$ represent the probability that the...
A manufacturer has three machine operators 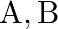 and
and 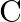 . The first operator
. The first operator 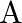 produces
produces 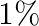 defective items, whereas the other two operators
defective items, whereas the other two operators  and
and  produce
produce  and
and 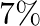 defective items respectively. A is on the job for
defective items respectively. A is on the job for 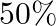 of the time,
of the time,  is on the job for
is on the job for 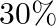 of the time and
of the time and 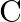 is on the job for
is on the job for 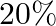 of the time. A defective item is produced, what is the probability that it was produced by A?
of the time. A defective item is produced, what is the probability that it was produced by A?
Solution: Let $\mathrm{E}_{1}$ be the event of machine time consumption. be the event of machine time consumption $\mathrm{A}, \mathrm{E}_{2}$ Let $B$ and $E_{3}$ represent the event of machine $C$...
Suppose a girl throws a die. If she gets a 5 or 6 , she tosses a coin three times and notes the number of heads. If she gets  or 4 , she tosses a coin once and notes whether a head or tail is obtained. If she obtained exactly one head, what is the probability that she threw
or 4 , she tosses a coin once and notes whether a head or tail is obtained. If she obtained exactly one head, what is the probability that she threw 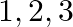 or 4 with the die?
or 4 with the die?
Solution: Let $\mathrm{E} {1}$ be the event where the die outcome is $5 or $6, $\mathrm{E} {2}$ be the event where the die outcome is $1,2,3$ or 4, and $A$ be the event where the die outcome is...
A factory has two machines  and
and  . Past record shows that machine A produced
. Past record shows that machine A produced 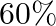 of the items of output and machine B produced
of the items of output and machine B produced  of the items. Further,
of the items. Further, 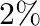 of the items produced by machine
of the items produced by machine  and
and 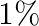 produced by machine
produced by machine  were defective. All the items are put into one stockpile and then one item is chosen at random from this and is found to be defective. What is the probability that it was produced by machine B?
were defective. All the items are put into one stockpile and then one item is chosen at random from this and is found to be defective. What is the probability that it was produced by machine B?
Solution: Let $E {1}$ represent the event that item A produces, $E {2}$ represent the event that item $B$ produces, and $X$ represent the event that the generated product is discovered to be...
An insurance company insured 2000 scooter drivers, 4000 car drivers and 6000 truck drivers. The probability of an accidents are 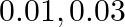 and
and  respectively. One of the insured persons meets with an accident. What is the probability that he is a scooter driver?
respectively. One of the insured persons meets with an accident. What is the probability that he is a scooter driver?
Solution: Let $\mathrm{E}_{1}$ represent the driver being a scooter driver, $\mathrm{E}_{2}$ represent the driver being a car driver, and $\mathrm{E}_{3}$ represent the driver being a truck driver....
There are three coins. One is a two-headed coin (having a head on both faces), another is a biased coin that comes up heads 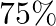 of the time and the third is an unbiased coin. One of the three coins is chosen at random and tossed, it shows heads, what is the probability that it was the two-headed coin?
of the time and the third is an unbiased coin. One of the three coins is chosen at random and tossed, it shows heads, what is the probability that it was the two-headed coin?
Solution: Let $E_{1}$ represent the event of selecting a two-headed coin, $E_{2}$ represent the event of selecting a biassed coin, and $\mathrm{E}_{3}$ represent the event of selecting an unbiased...
A laboratory blood test is 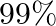 effective in detecting a certain disease when it is in fact, present. However, the test also yields a false-positive result for
effective in detecting a certain disease when it is in fact, present. However, the test also yields a false-positive result for 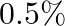 of the healthy person tested (i.e. if a healthy person is tested, then, with probability
of the healthy person tested (i.e. if a healthy person is tested, then, with probability 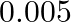 , the test will imply he has the disease). If
, the test will imply he has the disease). If  percent of the population actually has the disease, what is the probability that a person has the disease given that his test result is positive?
percent of the population actually has the disease, what is the probability that a person has the disease given that his test result is positive?
Solution: Let $\mathrm{E}_{1}$ represent a person who has a disease, $\mathrm{E}_{2}$ represent a person who does not have a disease, and $A$ represent a person who has a positive blood test....
In answering a question on a multiple choice test, a student either knows the answer or guesses. Let  be the probability that he knows the answer and
be the probability that he knows the answer and 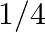 be the probability that he guesses. Assuming that a student who guesses at the answer will be correct with probability
be the probability that he guesses. Assuming that a student who guesses at the answer will be correct with probability 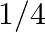 . What is the probability that the student knows the answer given that he answered it correctly?
. What is the probability that the student knows the answer given that he answered it correctly?
Solution: Let $E_{1}$ represent the situation in which the student knows the solution, $E_{2}$ represent the situation in which the student guesses the answer, and $\mathrm{A}$ represent the...
Of the students in a college, it is known that 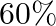 reside in hostel and
reside in hostel and  are day scholars (not residing in hostel). Previous year results report that
are day scholars (not residing in hostel). Previous year results report that 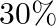 of all students who reside in hostel attain A grade and
of all students who reside in hostel attain A grade and 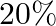 of day scholars attain A grade in their annual examination. At the end of the year, one student is chosen at random from the college and he has an A grade, what is the probability that the student is a hostlier?
of day scholars attain A grade in their annual examination. At the end of the year, one student is chosen at random from the college and he has an A grade, what is the probability that the student is a hostlier?
Solution: Let $\mathrm{E}_{1}$ represent the event that the student is a hostler, $\mathrm{E}_{2}$ represent the event that the student is a day scholar, and $A$ represent the event that the student...
A bag contains 4 red and 4 black balls, another bag contains 2 red and 6 black balls. One of the two bags is selected at random and a ball is drawn from the bag which is found to be red. Find the probability that the ball is drawn from the first bag.
Solution: Let $\mathrm{E}_{1}$ be the event of bag selection. The event of choosing the bag, say bag II, and the event of drawing a red ball are represented by $\mathrm{I}, \mathrm{E}_{2}$ and...
An urn contains 5 red and 5 black balls. A ball is drawn at random, its colour is noted, and is returned to the urn. Moreover, 2 additional balls of the colour drawn are put in the urn and then a ball is drawn at random. What is the probability that the second ball is red?
There are 5 red and 5 black balls in the urn. Let's say the ball drawn in the first attempt is red. $\Rightarrow P$ (probability of drawing a red ball) $=5 / 10=1 / 2$ The urn now holds 7 red and 5...
An urn contains 5 red and 2 black balls. Two balls are randomly drawn. Let 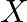 represent the number of black balls. What are the possible values of
represent the number of black balls. What are the possible values of 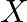 ? Is
? Is 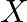 a random variable?
a random variable?
Solution: An urn containing 5 red and 2 black balls is presented. Let's call the red ball $mathrmR$ and the black ball $mathrmB$. Two balls are chosen at random. As a result, the experiment's sample...
If  and
and  are any two events such that
are any two events such that 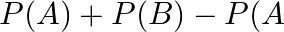 and
and 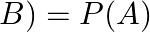 , then
, then
A. 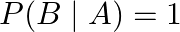
B. 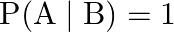
C. 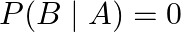
D. 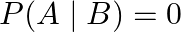
Solution: Answer: B. $P(A \mid B)=1$ Explanation: The following information is provided in the question: $A$ and $B$ are two examples of situations in which,Thus evaluating we have,$P(A)+P(B)-P(A$...
If 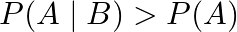 , then which of the following is correct: A.
, then which of the following is correct: A. 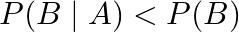
B. 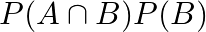
C. 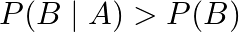
D. 
Solution: Answer: C. $P(B \mid A)>P(B)$Given in the question that, thus evaluating the value of parameters we have $P(A \mid B)>P(A)$ $\therefore \frac{P(A \cap B)}{P(B)}>P(A)$ $P(A \cap...
If  and
and  are two events such that
are two events such that 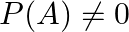 and
and 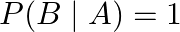 , then A.
, then A. 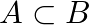
B. 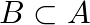
C. 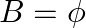
D.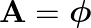
Solution: Answer: A. $A \subset B$ Explanation: The following information is provided in the question: $A$ and $B$ are two examples of situations in which, $P(A) \neq 0$ And, $\mathrm{P}(\mathrm{B}...
Bag I contains 3 red and 4 black balls and Bag II contains 4 red and 5 black balls. One ball is transferred from Bag I to Bag II and then a ball is drawn from Bag II. The ball so drawn is found to be red in colour. Find the probability that the transferred ball is black.
Solution: Let us first suppose that the events denoted by $A_1$ are the occurrences in which a red ball is transferred from bag I to bag II. In addition, $A_2$ denotes the occurrence in which a...
An electronic assembly consists of two subsystems, say, A and B. From previous testing procedures, the following probabilities are assumed to be known: 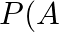 fails
fails 
 B fails alone)
B fails alone) 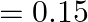
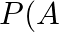 and
and  fail
fail 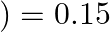
Evaluate the following probabilities: (i) P (A fails | B has failed)
(ii) P (A fails alone)
Solution: (i) Take, for example, the event that is failed by $A$, which is symbolised by the symbol $E A$. Furthermore, an event that is failed by $B$ is marked by the symbol $E B$. The following...
If each element of a second order determinant is either zero or one, what is the probability that the value of the determinant is positive? (Assume that the individual entries of the determinant are chosen independently, each value being assumed with probability 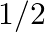
Solution: We can deduce the following information from the question: The total number of determinants of second order in which the element is or is not $1=(2)^{4}$ $=16$ Now, we have the value of...
Assume that the chances of a patient having a heart attack are  . It is also assumed that a meditation and yoga course reduce the risk of heart attack by
. It is also assumed that a meditation and yoga course reduce the risk of heart attack by 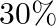 and prescription of certain drug reduces its chances by
and prescription of certain drug reduces its chances by 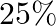 . At a time a patient can choose any one of the two options with equal probabilities. It is given that after going through one of the two options the patient selected at random suffers a heart attack. Find the probability that the patient followed a course of meditation and yoga?
. At a time a patient can choose any one of the two options with equal probabilities. It is given that after going through one of the two options the patient selected at random suffers a heart attack. Find the probability that the patient followed a course of meditation and yoga?
Solution: Let us suppose that $X$ represents the events that caused a person to have a heart attack. $A_1$ denotes events in which the selected person has followed the course of yoga and meditation...
Suppose we have four boxes A, B, C and D containing coloured marbles as given below: One of the boxes has been selected at random and a single marble is drawn from it. If the marble is red, what is the probability that it was drawn from box  ?, box B?, box C?
?, box B?, box C?
Solution: Let us assume $R$ be the event of drawing the red marblesLet us additionally assume that the letters $E_{A}, E_{B}$ and $E_{c}$ denote the boxes A, B and C respectively Given that, Total...
In a game, a man wins a rupee for a six and loses a rupee for any other number when a fair die is thrown. The man decided to throw a die thrice but to quit as and when he gets a six. Find the expected value of the amount he wins / loses.
For the situation given in the equation, we have Probability of getting a six in a throw of a die $=1 / 6$ Also, probability of not getting a $6=5 / 6$ Following that, there are three scenarios from...
How many times must a man toss a fair coin so that the probability of having at least one head is more than  ?
?
Solution: Let us suppose that a man throws the coin $n$ times before calling a winner. As a result, the $n$ tosses are the Bernoulli trials.$\therefore$ Probability of getting head at the toss of...
An experiment succeeds twice as often as it fails. Find the probability that in the next six trials, there will be at least 4 successes.
Solution: Given that probability of failure $=x$ And, probability of success $=2 x$ $\therefore x+2 x=1$ $3 x=1$ $x=1 / 3$ $2 x=2 / 3$ Let $p=1 / 3$ and $q=2 / 3$ In addition, let $X$ be the random...
If a leap year is selected at random, what is the chance that it will contain 53 Tuesdays?
Solution: We know that, in a leap year there are total 366 days, 52 weeks and 2 days Now, in 52 weeks there are total 52 Tuesdays $\therefore$ When a leap year contains 53 Tuesdays, the likelihood...
A die is thrown again and again until three sixes are obtained. Find the probability of obtaining the third six in the sixth throw of the die.
Solution: It is apparent from the question that was posed that The probabiltiy of getting a six on a single die throw $=1 / 6$ And, probability of not getting a six $=5 / 6$ Let's assume, $p=1 / 6$...
In a hurdle race, a player has to cross 10 hurdles. The probability that he will clear each hurdle is 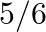 . What is the probability that he will knock down fewer than 2 hurdles?
. What is the probability that he will knock down fewer than 2 hurdles?
Solution: Assume that $p$ represents the chance of a player clearing the hurdle and $q$ represents the likelihood of a player knocking down the hurdle. $\therefore p=5 / 6$ and $q=1-5 / 6=1 / 6$ Let...
An urn contains 25 balls of which 10 balls bear a mark ‘ 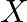 ‘ and the remaining 15 bear a mark ‘Y’. A ball is drawn at random from the urn, its mark is noted down and it is replaced. If 6 balls are drawn in this way, find the probability that: (i) At least one ball will bear ‘
‘ and the remaining 15 bear a mark ‘Y’. A ball is drawn at random from the urn, its mark is noted down and it is replaced. If 6 balls are drawn in this way, find the probability that: (i) At least one ball will bear ‘ 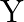 ‘ mark.
‘ mark.
(ii) The number of balls with ‘ 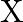 ‘ mark and ‘
‘ mark and ‘ 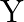 ‘ mark will be equal.
‘ mark will be equal.
Given in the question that, Total number of balls in the urn $=25$ Number of balls bearing mark ${ }^{\prime} \mathrm{X}^{\prime}=10$ Number of balls bearing mark ${ }^{\prime}...
An urn contains 25 balls of which 10 balls bear a mark ‘ 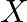 ‘ and the remaining 15 bear a mark ‘Y’. A ball is drawn at random from the urn, its mark is noted down and it is replaced. If 6 balls are drawn in this way, find the probability that: (i) All will bear ‘
‘ and the remaining 15 bear a mark ‘Y’. A ball is drawn at random from the urn, its mark is noted down and it is replaced. If 6 balls are drawn in this way, find the probability that: (i) All will bear ‘ 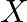 ‘ mark.
‘ mark.
(ii) Not more than 2 will bear ‘Y’ mark.
Solution:(i) Given in the question that, Total number of balls in the urn $=25$ Number of balls bearing mark ${ }^{\prime} \mathrm{X}^{\prime}=10$ Number of balls bearing mark ${ }^{\prime}...
Suppose that  of people are right-handed. What is the probability that at most 6 of a random sample of 10 people are right-handed?
of people are right-handed. What is the probability that at most 6 of a random sample of 10 people are right-handed?
Solution: Given that, $90 \%$ of the people are right handed Let $p$ represent the probability of right-handed people and $q$ represent the probability of left-handed people. $p=9 / 10$ and $q=1-9 /...
Suppose that  of men and
of men and 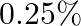 of women have grey hair. A grey haired person is selected at random. What is the probability of this person being male? Assume that there are equal number of males and females.
of women have grey hair. A grey haired person is selected at random. What is the probability of this person being male? Assume that there are equal number of males and females.
Solution: Given that, $5 \%$ of men and $0.25 \%$ of women have grey hair $\therefore$ Total $\%$ of people having grey hair $=5+0.25$ $=5.25 \%$ Hence, the Probability of having a selected person...
A couple has two children, (i) Find the probability that both children are males, if it is known that at least one of the children is male.
(ii) Find the probability that both children are females, if it is known that the elder child is a female.
Solution:(i) According to the question, if the couple has two children then the sample space is: $S={(b, b),(b, g),(g, b),(g, g)}$ Assume A denotes the event of both children having male children,...
 and
and  are two events such that
are two events such that 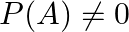 . Find
. Find 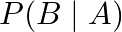 , if:
, if:
(i)  is a subset of
is a subset of 
(ii) 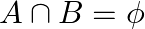
Solution:It is given that,$A$ and $B$ are two events such that $P(A) \neq 0$We have, $A \cap B=A$$$\begin{aligned}&\therefore P(A \cap B)=P(B \cap A)=P(A) \&\text { Hence, } P(B \mid...
A container contains 24 marbles, some are green and others are blue. In the event that a marble is drawn at arbitrary from the container, the likelihood that it is green is ⅔. Track down the quantity of blue balls n the container.
Solution: Absolute marbles = 24 Let the absolute green marbles = x Along these lines, the complete blue marbles = 24-x P(getting green marble) = x/24 From the inquiry, x/24 = ⅔ Along these lines,...
A crate contains 12 balls out of which x are dark. On the off chance that one ball is drawn indiscriminately from thebox, what is the likelihood that it will be a debase?On the off chance that 6 more torpedoes are placed in the case, the likelihood of drawing a renounce is presentlytwofold of what it was previously. Discover x
Solution: All out number of debases = x All out number of balls = 12 P(E) = (Number of positive results/Total number of results) P (getting debases) = x/12 — — — - (I) Presently, when 6 more debases...
A sack contains 5 red balls and some blue balls. In the event that the likelihood of drawing a blue ball is twofold that of a red ball, decide the quantity of blue balls taken care of.
Solution: It is given that the absolute number of red balls = 5 Let the all out number of blue balls = x In this way, the all out no. of balls = x+5 P(E) = (Number of good results/Total number of...
A pass on is numbered so that its faces show the numbers 1, 2, 2, 3, 3, 6. It is tossed multiple times and the complete score in two tosses is noted. Complete the accompanying table which gives a couple of upsides of the absolute score on the two tosses:
What is the likelihood that the absolute score is (I) even? (ii) 6? (iii) something like 6? Solution: The table will be as per the following: 1 2 2 3 3 6 1 2 3 3 4 4 7 2 3 4 4 5 5 8 2 3 4 4 5 5 8 3...
Two clients Shyam and Ekta are visiting a specific shop around the same time (Tuesday to Saturday). Each is similarly prone to visit the shop on any day as on one more day. What is the likelihood that both will visit the shop on
(I) that very day? (ii) back to back days? (iii) distinctive days? Solution: Since there are 5 days and both can go to the shop in 5 different ways each thus, The complete number of potential...
A stash contains hundred 50p coins, fifty ₹1 coins, twenty ₹2 coins and ten ₹5 coins. In case almost certainly, one of the coins will drop out when the bank is flipped around, what is the likelihood that the coin
(I) will be a 50 p coin? (ii) won't be a ₹5 coin? Solution: Complete no. of coins = 100+50+20+10 = 180 P(E) = (Number of ideal results/Total number of results) (I) Total number of 50 p coin = 100 P...
Gopi purchases a fish from a shop for his aquarium. The retailer takes out one fish indiscriminately from a tank containing 5 male fish and 8 female fish (see Fig. 15.4). What is the likelihood that the fish taken out is a male fish?
Solution: The all out number of fish in the tank = 5+8 = 13 All out number of male fish = 5 P(E) = (Number of good results/Total number of results) P (male fish) = 5/13 = 0.38
A shot in the dark comprises of turning a bolt which stops pointing at one of the numbers 1, 2, 3, 4, 5, 6, 7, 8 (see Fig. 15.5), and these are similarly probable results. What is the likelihood that it will point at
(I) 8? (ii) an odd number? (iii) a number more noteworthy than 2? (iv) a number under 9? Solution: Complete number of potential results = 8 P(E) = (Number of great results/Total number of results)...
The probability that a student is not a swimmer is 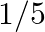 . Then the probability that out of five students, four are swimmers is
. Then the probability that out of five students, four are swimmers is
A. 
B. 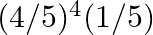
C. 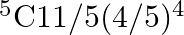
D. None of these
Solution:Answer: A. ${ }^{5} \mathrm{C}_{4} 1 / 5(4 / 5)^{4}$ Explanation: Assume that $X$ is the number of pupils who are swimmers out of a class of five. The Bernoulli trials are also used to...
A bite the dust is tossed once. Discover the likelihood of getting
(I) an indivisible number; (ii) a number lying somewhere in the range of 2 and 6; (iii) an odd number. Solution: Complete potential occasions when a dice is tossed = 6 (1, 2, 3, 4, 5, and 6) P(E) =...
In a box containing 100 bulbs, 10 are defective. The probability that out of a sample of 5 bulbs, none is defective is A. 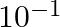
B. 
C. 
D. 
Solution:Answer: C. $(9 / 10)^{5}$ Explanation: Assume $X$ is the number of times a random sample of 5 bulbs was selected for faulty bulbs. Bernoulli trials are also the process of repeatedly...
One card is drawn from a very much rearranged deck of 52 cards. Discover the likelihood of getting
(I) a lord of red tone (ii) a face card (iii) a red face card (iv) the jack of hearts (v) a spade (vi) the sovereign of jewels Arrangement: All out number of potential results = 52 P(E) = (Number of...
Five cards the ten, jack, sovereign, ruler and trick card, are very much rearranged with their face downwards. One card is then gotten up.
(I) What is the likelihood that the card is the sovereign? (ii) If the sovereign is drawn and set to the side, what is the likelihood that the subsequent card gotten is (a) an ace? (b) a sovereign?...
12 flawed pens are inadvertently blended in with 132 great ones. It is unimaginable to simply take a gander at a pen and tell whether it is blemished. One pen is taken out aimlessly from this parcel. Decide the likelihood that the pen taken out is a decent one.
Solution: Quantities of pens = Numbers of blemished pens + Numbers of good pens ∴ Total number of pens = 132+12 = 144 pens P(E) = (Number of great results/Total number of results) P(picking a decent...
It is known that 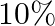 of certain articles manufactured are defective. What is the probability that in a random sample of 12 such articles, 9 are defective?
of certain articles manufactured are defective. What is the probability that in a random sample of 12 such articles, 9 are defective?
Solutions: Assume that $X$ is the number of times a defective article was selected from a random sample space of 12 articles. Bernoulli trials are also repeated articles in a random sample space....
(I) A ton of 20 bulbs contain 4 blemished ones. One bulb is drawn indiscriminately from the part. What is the likelihood that this bulb is damaged?(ii) Suppose the bulb attracted (I) isn’t damaged and isn’t supplanted. Presently one bulb is drawn indiscriminately from the rest. What is the likelihood that this bulb isn’t deficient?
Solution: (I) Number of damaged bulbs = 4 The absolute number of bulbs = 20 P(E) = (Number of great results/Total number of results) ∴ Probability of getting a damaged bulb = P (deficient bulb) =...
Find the probability of throwing at most 2 sixes in 6 throws of a single die.
Solution: Let's say $X$ represents the amount of times you've gotten sixes in six die throws. Bernoulli trials are also the repeated tossing of die selection. As a result, the chances of getting six...
Find the probability of getting 5 exactly twice in 7 throws of a die.
Solutions: Let's say $X$ represents the number of times you've gotten 5 in 7 die throws. The Bernoulli trials are also the repeated tossing of a die. Thus, probability of getting 5 in a single...
A container contains 90 plates which are numbered from 1 to 90. On the off chance that one circle is drawn aimlessly from the crate, discover the likelihood that it bears
(I) a two-digit number (ii) an ideal square number (iii) a number distinguishable by 5. Solution: The complete number of plates = 90 P(E) = (Number of great results/Total number of results) (I)...
A kid has a kick the bucket whose six faces show the letters as given underneath:
The bite the dust is tossed once. What is the likelihood of getting (I) A? (ii) D? Solution: The complete number of potential results (or occasions) = 6 P(E) = (Number of ideal results/Total number...
A person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is 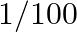 . What is the probability that he will win a prize
. What is the probability that he will win a prize
(a) At least once
(b) Exactly once
(c) At least twice?
Solution: (a) Let $X$ be the number of prizes won in 50 lottery drawings, and the trials be Bernoulli trials.Here clearly, we have $\mathrm{X}$ is a binomial distribution where $\mathrm{n}=50$ and...
Assume you drop a kick the bucket aimlessly on the rectangular locale displayed in Fig. 15.6. What is the likelihood that it will land inside the circle with breadth 1m?
Solution: To start with, compute the space of the square shape and the space of the circle. Here, the space of the square shape is the conceivable result and the space of the circle will be the...
On a multiple choice examination with three possible answers for each of the five questions, what is the probability that a candidate would get four or more correct answers just by guessing?
Solution: Bernoulli trials are the repeated correct answer guessing from the given multiple choice questions in this question. Assume that $X$ represents the number of correct answers in the...
Suppose 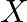 has a binomial distribution
has a binomial distribution 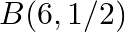 . Show that
. Show that 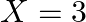 is the most likely outcome. (Hint:
is the most likely outcome. (Hint: 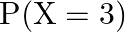 is the maximum among all
is the maximum among all 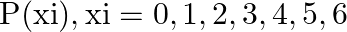 )
)
Solution: Given $\mathrm{X}$ is any random variable whose binomial distribution is $\mathrm{B}(6,1 / 2)$ Thus, $\mathrm{n}=6$ and $\mathrm{p}=1 / 2$ $q=1-p=1-1 / 2=1 / 2$ Thus, $P(X=x)={ }^{n} C_{x}...
A great deal comprises of 144 ball pens of which 20 are deficient and the others are acceptable. Nuri will purchase a pen in case it is acceptable, yet won’t accepting in case it is damaged. The retailer draws one pen indiscriminately and offers it to her. What is the likelihood that
(I) She will get it? (ii) She won't get it? Arrangement: The all out quantities of results for example pens = 144 Given, quantities of inadequate pens = 20 ∴ The quantities of non inadequate pens =...
In an examination, 20 questions of true-false type are asked. Suppose a student tosses a fair coin to determine his answer to each question. If the coin falls heads, he answers ‘true’; if it falls tails, he answers ‘false’. Find the probability that he answers at least 12 questions correctly.
Solution: Assume that $x$ is the number of correctly answered questions out of a total of twenty. Since the 'head' of the coin represents the correct answer and the 'tail' represents the incorrect...
(I) Complete the accompanying table: (ii) An understudy contends that ‘there are 11 potential results 2, 3, 4, 5, 6, 7, 8, 9, 10, 11 and 12. In this way, every one of them has a likelihood 1/11. Do you concur with this contention? Legitimize your Solution:.
Solution: On the off chance that 2 dices are tossed, the potential occasions are: (1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6) (2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6) (3, 1), (3, 2), (3,...
A bag consists of 10 balls each marked with one of the digits 0 to 9. If four balls are drawn successively with replacement from the bag, what is the probability that none is marked with the digit 0?
Solution: Assume that the number of balls with a digit marked as zero in the experiment of four balls drawn at the same time is $x$. The trial is a Bernoulli trial because the balls are drawn with...
A game comprises of throwing a one rupee coin multiple times and taking note of its result each time. Hanif wins if every one of the throws give a similar outcome i.e., three heads or three tails, and loses in any case. Ascertain the likelihood that Hanif will lose the game.
Solution: The absolute number of results = 8 (HHH, HHT, HTH, THH, TTH, HTT, THT, TTT) Absolute results in which Hanif will lose the game = 6 (HHT, HTH, THH, TTH, HTT, THT) P (losing the game) = 6/8...
The probability that a bulb produced by a factory will fuse after 150 days of use is 0.05. Find the probability that out of 5 such bulbs
(i) more than one
(ii) at least one will fuse after 150 days of use.
Solution: Assume that in a five-trial experiment, the number of bulbs that will fuse after 150 days is $x$. The trials will be Bernoulli trials, as we can see, because they are made with...
The probability that a bulb produced by a factory will fuse after 150 days of use is 0.05. Find the probability that out of 5 such bulbs
(i) none
(ii) not more than one
Solution: Assume that in a five-trial experiment, the number of bulbs that will fuse after 150 days is $x$. The trials will be Bernoulli trials, as we can see, because they are made with...
A bite the dust is tossed twice. What is the likelihood that
(I) 5 won't come up one or the other time? (ii) 5 will come up in some measure once? [Hint : Throwing a bite the dust twice and tossing two dice at the same time are treated as the equivalent...
Five cards are drawn successively with replacement from a well-shuffled deck of 52 cards. What is the probability that
(i) All the five cards are spades?
(ii) Only 3 cards are spades?
(iii) None is a spade?
Solution: Let's say there are $x$ spade cards among the five drawn cards. Because we can see that the cards are being drawn with replacement, the trials will be Bernoulli trials. We now know that...
Which of the accompanying contentions are right and which are not right? Give purposes behind your Solution:.
(I) If two coins are thrown at the same time there are three potential results—two heads, two tails or one of each. In this manner, for every one of these results, the likelihood is 1/3 (ii) If a...
There are 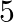 defective items in a large bulk of items. What is the probability that a sample of 10 items will include not more than one defective item?
defective items in a large bulk of items. What is the probability that a sample of 10 items will include not more than one defective item?
Solution: Let's say there are $x$ defective items in a sample of ten drawn sequentially. As we can see, the items' drawings are now done with replacement. As a result, the trials are Bernoulli...
A marble is picked out at random from a box containing 5 red marbles, 8 white marbles and 4 green marbles. What is the probability that the marble picked out will be
(i) a red marble? (ii) a white marble? (iii) is not a green marble? Solution: The Total number of marbles in the box = 5+8+4 = 17 P(E) = (Number of favourable outcomes/ Total number of outcomes) (i)...
A ball is picked at random from a bag containing 3 red balls and 5 black balls. What is the probability that the ball picked up at random is
(i) a red ball? (ii) not a red ball? Solution: The total number of balls inside the bag = Number of red balls + Number of black balls So, the total no. of balls inside the bag = 5+3 = 8 As we know...
In a group of 3 students, it is given that the probability of 2 students not having the same birth date is 0.992. What is the probability that the 2 students have the same birth date?
Solution: Let E be the event wherein 2 students having the same birth date. A/Q Given, P(E) = 0.992 As we know, P(E)+P(not E) = 1 So, P(not E) = 1–0.992 = 0.008 ∴ The probability that the 2 students...
A bag contains only lemon flavored candies. Malini picked one candy from the bag without looking into it. What is the probability that she picked up
(i) a candy of orange flavor? (ii) a candy of lemon flavored? Solution: (i) As we know that the bag contains lemon-flavored candies only. So, The no. of orange flavored candies = 0 So, the...
If P(E) = 0.05, what is the probability of P(not E)?
Solution: We know that, P(E)+P(not E) = 1 A/Q It is given that, P(E) = 0.05 So, P(not E) = 1-P(E) Or, P(not E) = 1-0.05 ∴ P(not E) = 0.95
Which of the below mentioned values cannot be the probability of an event?
(A) 2/3 (B) -1.5 (C) 15% (D) 0.7 Solution: The probability of any event (E) must lie between 0 and 1 i.e. 0 ≤ P(E) ≤ 1. So, from the above options, only option (B) -1.5 cannot be the probability of...
There are  defective items in a large bulk of items. What is the probability that a sample of 10 items will include not more than one defective item?
defective items in a large bulk of items. What is the probability that a sample of 10 items will include not more than one defective item?
Solution: Let's say there are $x$ defective items in a sample of ten drawn sequentially. As we can see, the items' drawings are now done with replacement. As a result, the trials are Bernoulli...
A pair of dice is thrown 4 times. If getting a doublet is considered a success, find the probability of two successes.
Solution: Bernoulli trials are defined as the repeated tosses of a pair of dice. Let $x$ be the number of times you get doublets in an experiment where you throw two dice at the same time four...
Why tossing a coin is considered to be a fair way of deciding which team should get the ball at the beginning of a football game?
Solution: Coin tossing is a fair way of deciding because the number of possible outcomes are only 2 i.e. either it is a head or a tail. Since these two outcomes are an equally likely outcome,...
A die is thrown 6 times. If ‘getting an odd number’ is a success, what is the probability of at most 5 successes?
Solution: Bernoulli trials are known for their repeated tosses of a dice. Let $x$ be the number of times an odd number was obtained in a six-trial experiment. Probability of getting at most 5...
Which of the following experiments mentioned below have equally likely outcomes? Explain.
(i) A driver attempts to start a car. The car starts or does not start. (ii) A player tries to shoot a basketball. She/he shoots perfectly or misses the shot. (iii) A trial is made to a Solution: a...
A die is thrown 6 times. If ‘getting an odd number’ is a success, what is the probability of
(i) 5 successes?
(ii) At least 5 successes?
Solution: Bernoulli trials are known for their repeated tosses of a dice. Let $x$ be the number of times an odd number was obtained in a six-trial experiment.Odds of getting an odd number in a...
Complete the statements given below:-
(i) The probability for any event E, P(E) + P(not E) ___________. (ii) The probability of an event that will never occur is __________. These events are called ________. (iii) The probability of an...
An urn contains 5 red and 5 black balls. A ball is drawn at random, its colour is noted and is returned to the urn. Moreover, 2 additional balls of the colour drawn are put in the urn and then a ball is drawn at random. What is the probability that the second ball is red?
Solution: Given: Urn contains 5 red and 5 black balls. Let in first attempt the ball drawn is of red colour. $\Rightarrow P$ (probability of drawing a red ball) $=5 / 10=1 / 2$ After the two balls...
Two events  and
and  will be independent, if
will be independent, if
(A) 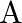 and
and 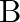 are mutually exclusive
are mutually exclusive
(B) ![Rendered by QuickLaTeX.com P\left(A^{\prime} B^{\prime}\right)=[1-P(A)][1-P(B)]](https://www.learnatnoon.com/s/wp-content/ql-cache/quicklatex.com-ed48ea5f986832c34caa5efbac8759a1_l3.png)
(C) 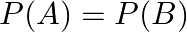
(D) 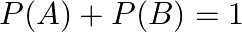
Solution: Answer: (B) $P\left(A^{\prime} B^{\prime}\right)=[1-P(A)][1-P(B)]$Concept: Two events are independent, statistically independent, or stochastically independent if the occurrence of one...
The probability of obtaining an even prime number on each die, when a pair of dice is rolled is
A. 0
B. 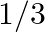
c. 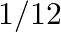
D. 
Solution: Answer: D. $1 / 36$ Explanation: Given A pair of dice is rolled. Hence the number of outcomes $=36$ The chance of getting an even prime number on each die is represented by the...
In a hostel, 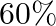 of the students read Hindi newspaper,
of the students read Hindi newspaper,  read English newspaper and
read English newspaper and 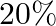 read both Hindi and English newspapers. A student is selected at random.
read both Hindi and English newspapers. A student is selected at random.
(a) Find the probability that she reads neither Hindi nor English newspapers.
(b) If she reads Hindi newspaper, find the probability that she reads English newspaper.
(c) If she reads English newspaper, find the probability that she reads Hindi newspaper
Given: The letters $H$ and $E$ stand for the number of students who read the Hindi newspaper and the English daily, respectively. Hence, $\mathrm{P}(\mathrm{H})=$ Probability of students who read...
One card is drawn at random from a well shuffled deck of 52 cards. In which of the following cases are the events  and
and  independent?
independent?
(i) E: ‘the card drawn is a spade’ F: ‘the card drawn is an ace’
(ii) E: ‘the card drawn is black’  : ‘the card drawn is a king’
: ‘the card drawn is a king’
(iii) E: ‘the card drawn is a king or queen’ F: ‘the card drawn is a queen or jack’.
Solution: Given: A deck of 52 cards. Concept: Two events are independent, statistically independent, or stochastically independent if the occurrence of one does not affect the probability of...
Probability of solving specific problem independently by 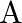 and
and 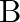 are
are 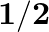 and
and 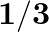 respectively. If both try to solve the problem independently, find the probability that (i) The problem is solved
respectively. If both try to solve the problem independently, find the probability that (i) The problem is solved
(ii) Exactly one of them solves the problem.
Given, $P(A)=$ Probability of solving the problem by $A=1 / 2$ Concept: $\mathrm{P}(\mathrm{B})=$ Probability of solving the problem by $\mathrm{B}=1 / 3$ Because $A$ and $B$ both are...
Two balls are drawn at random with replacement from a box containing 10 black and 8 red balls. Find the probability that (i) both balls are red.
(ii) First ball is black and second is red.
(iii) One of them is black and other is red.
Solution: Given A box with ten black and eight red balls. Total number of balls in box = 18 (i) Both balls are red. Probability of getting a red ball in first draw $=8 / 18=4 / 9$ As the ball is...
A die is tossed thrice. Find the probability of getting an odd number at least once.
Given: A die is tossed three times. Then the sample space $S={1,2,3,4,5,6}$ Let $\mathrm{P}(\mathrm{A})=$ probability of getting an odd number in first throw. $\Rightarrow P(A)=3 / 6=1 / 2 .$ Let...
Events  and
and  are such that
are such that 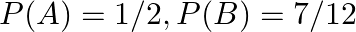 and
and  not
not  or
or 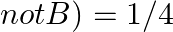 . State whether
. State whether  and
and  are independent?
are independent?
Solution: Given: $P(A)=1 / 2, P(B)=7 / 12$ and $P($ not $A$ or $\operatorname{not} B)=1 / 4$Concept: Two events are independent, statistically independent, or stochastically independent if the...
If  and
and  are two events such that
are two events such that  and
and 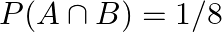 , find
, find  not
not  and not B).
and not B).
Solution: Given $P(A)=1 / 4, P(B)=1 / 2$ and $P(A \cap B)=1 / 8$ Concept: $P($ not $A$ and $\operatorname{not} B)=P\left(A^{\prime} \cap B^{\prime}\right)$ As, $\left{A^{\prime} \cap B^{\prime}=(A...
Let  and
and  be independent events with
be independent events with 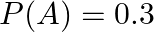 and
and 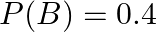 . Find (i)
. Find (i) 
(ii) 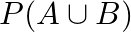
(iii) 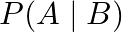
(iv) 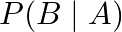
Solution: Given: $\mathrm{P}(\mathrm{A})=0.3$ and $\mathrm{P}(\mathrm{B})=0.4$ (i) $P(A \cap B)$Given $P ( A )=0.3$ and $P ( B )=0.4$ When A and B are independent.Two events are independent,...
Let  and
and  be independent events with
be independent events with 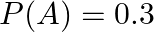 and
and 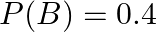 . Find (i)
. Find (i) 
(ii) 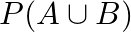
(iii) 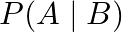
(iv) 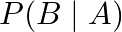
Given $P ( A )=0.3$ and $P ( B )=0.4$ (i) $P(A \cap B)$ When A and B are independent. Two events are independent, statistically independent, or stochastically independent if the occurrence of one...
Given that the events  and
and  are such that
are such that 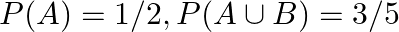 and
and 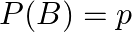 . Find
. Find 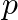 if they are (i) mutually exclusive (ii) independent.
if they are (i) mutually exclusive (ii) independent.
Solution: Given: $\mathrm{P}(\mathrm{A})=1 / 2, \mathrm{P}(\mathrm{A} \cup \mathrm{B})=1 / 5$ and let the probability of event be $\mathrm{P}(\mathrm{B})=\mathrm{p}$ (i) Mutually exclusive When A...
Let  and
and 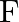 be events with
be events with 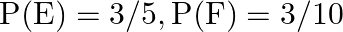 and
and 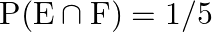 . Are
. Are  and
and 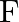 independent?
independent?
Solution: Given: $P(E)=3 / 5, P(F)=3 / 10$ and $P(E \cap F)=1 / 5$Evaluating the value of parameter & comparing with given, $P(E) . P(F)=3 / 5 \times 3 / 10=9 / 50 \neq 1 / 5$ $\Rightarrow P(E...
A die marked 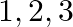 in red and
in red and 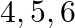 in green is tossed. Let
in green is tossed. Let  be the event, ‘the number is even,’ and
be the event, ‘the number is even,’ and  be the event, ‘the number is red’. Are A and B independent?
be the event, ‘the number is red’. Are A and B independent?
Solution: The dice sample space will be be $S={1,2,3,4,5,6}$ Let $A$ be the event, the number is even, sample space of the event: $\Rightarrow \mathrm{A}={2,4,6}$ $\Rightarrow P(A)=3 / 6=1 / 2$ Now,...
A fair coin and an unbiased die are tossed. Let A be the event ‘head appears on the coin’ and 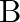 be the event
be the event 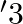 on the die’. Check whether
on the die’. Check whether 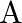 and
and 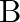 are independent events or not.
are independent events or not.
Given: A fair coin and an unbiased die are tossed. Let A be the event head appears on the coin. So the sample space of the event will be: $\Rightarrow A={(\mathrm{H}, 1),(\mathrm{H}, 2),(\mathrm{H},...
A box of oranges is inspected by examining three randomly selected oranges drawn without replacement. If all the three oranges are good, the box is approved for sale, otherwise, it is rejected. Find the probability that a box containing 15 oranges out of which 12 are good and 3 are bad ones will be approved for sale.
Solution: Given: A box of oranges. Let A, B, and C represent the events that occur when the first, second, and third drawn oranges are all excellent. Now, $P(A)=P$ (good orange in first draw) $=12 /...
Two cards are drawn at random and without replacement from a pack of 52 playing cards. Find the probability that both the cards are black.
Given: A pack of 52 cards. As we all know, there are a total of 26 black cards. Let $A$ and $B$ represent the first and second drawn cards being black, respectively. Now if the probability of event...
If 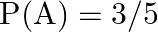 and
and  , find
, find 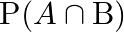 if
if 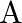 and
and 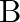 are independent events.
are independent events.
Solution: Given: $P(A)=3 / 5$ and $P(B)=1 / 5$ As A and B are independent events.So we know that for independent events,$\Rightarrow P(A \cap B)=P(A) . P(B)$Substituiting the values we get, $=3 / 5...
If 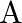 and
and 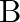 are events such that
are events such that 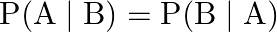 , then
, then
A. 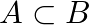 but
but 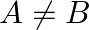
B. 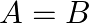
C. 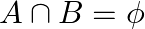
D. 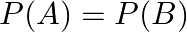
Solution:Answer: D. $P(A)=P(B)$ Explanation: Given: $\mathrm{P}(\mathrm{A} \mid \mathrm{B})=\mathrm{P}(\mathrm{B} \mid \mathrm{A})$We are aware that, according to the concept of conditional...
If 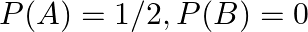 , then
, then 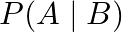 is
is
A. 0
B. 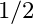
C. not defined
D. 1
Answer: C. Not defined Explanation: We are aware that, according to the concept of conditional probability, $\mathrm{P}(\mathrm{A} \mid \mathrm{B})=\frac{\mathrm{P}(\mathrm{A} \cap...
Consider the experiment of throwing a die, if a multiple of 3 comes up, throw the die again and if any other number comes, toss a coin. Find the conditional probability of the event ‘the coin shows a tail’, given that ‘at least one die shows a 3 ‘.
Let $E$ be the event that 'the coin shows a tail' and $F$ be the event that 'at least one die shows a 3 '. $\Rightarrow \mathrm{E}={1 \mathrm{~T}, 2 \mathrm{~T}, 4 \mathrm{~T}, 5 \mathrm{~T}}$ and...
Consider the experiment of throwing a die, if a multiple of 3 comes up, throw the die again and if any other number comes, toss a coin. Find the conditional probability of the event ‘the coin shows a tail’, given that ‘at least one die shows a 3 ‘.
Let $E$ be the event that 'the coin shows a tail' and $F$ be the event that 'at least one die shows a 3 '. $Rightarrow mathrm{E}={1 mathrm{~T}, 2 mathrm{~T}, 4 mathrm{~T}, 5 mathrm{~T}}$ and...
